Adaptable Curricular Exercises (ACE) for Quantum Information Science (QIS)
Our goals for this site are to provide adaptable resources to support faculty, including those very new to these topics, who wish to add some student-centered, research-based QIS instruction to your classes.
We have materials for two different audiences, depending on the type of course you teach.
QM Course: Are you teaching quantum mechanics in a physics department to (mostly) majors, and want to add a short unit on quantum information (e.g. quantum computing or cryptography) within your course?

QIS Course: Are you teaching a quantum computing/QIS course to a diverse population of students (e.g. computer science, physics, engineering, math...), and want to support students in the basic quantum mechanics needed?
(These materials are under development)
And: if you teach undergraduate quantum mechanics to physics students and are looking for a suite of student-centered materials to help you with the entire QM course, please visit our Adaptable curricular materials for Quantum Mechanics site.
Tools for introducing QIS in a QM course
Who is this for? An instructor teaching Quantum Mechanics who would like to add some quantum information science topics to their physics course.
(Materials are suitable for faculty new to these topics.)
Topics: We have divided our materials into three main topical areas.
Each is standalone - materials can be taught without any prerequisite material from the other groups.
(Because of this, there are some duplicate materials across the groups.)
We do not intend for the materials to be used wholly as written. Rather, they form a complete set that a faculty member can pick and choose from as it fits their schedule, the material already taught, and the interests of both the faculty member and student.
Types of instructional materials
The “instructional materials” found on this site include: lecture notes for faculty, concept tests, homework questions, online tutorials, and assessments.
Please use and adapt whatever is helpful to you, however it will most benefit your students. Please credit our work if you share your materials beyond your own classes. Please make an effort to keep assessment materials off the open web - alter questions for your students.
 Example Classroom Implementations
Example Classroom Implementations
Curriculum designers Dr. Steven Pollock and Dr. Gina Passante have each taught this material in their classes. Feel free to download our lecture notes - we are happy for you to use whatever material works for your class.
Please email if you try our materials and have any feedback:
steven.pollock (at) colorado.edu or gpassante (at) fullerton.edu
Steven Pollock's CU Boulder Implementation
CUB is a research-intensive public institution. I teach these materials in junior or senior-level undergraduate quantum mechanics courses, which currently all follow a spins-first curriculum (using McIntyre's Quantum Mechanics textbook.)
Each sequence below takes me one week. These are all taught in "large lecture" (75+ student) settings with three 50-minute lectures/week.
- I teach entanglement and EPR midway through the first semester (this is largely following McIntyre's Chapter 4 treatment)
- I teach qubits and teleportation late in the second semester (senior-level) quantum course, as a basic introduction to quantum information science. (This is largely following McIntyre's Chapter 16 treatment)
Please reach out if you have questions or feedback: steven.pollock (at) colorado.edu
Gina Passante's CSUF Implementation
CSUF is a primarily undergraduate, large, Hispanic-serving institution. I teach these materials in several of my quantum mechanics courses at the undergraduate and master's levels.
- Qubits, Quantum Gates, and Teleportation: I choose to teach this in the middle of a first semester of undergraduate QM. My course is spins-first and I do a "quantum computing" week to apply what they have learned before moving to position wave functions.
- Entanglement and EPR: I teach an introduction to entanglement and a conversation of "spooky action at a distance" when I teach the "qubits, quantum gates, and teleportation" material. But the discussion about the EPR paradox and the Bell inequalities is taught at the master's level.
- Quantum Cryptography: This is a one-class supplement that can be taught at any level. The only prerequisite knowledge is superposition states, measurement, and bra-ket notation. The example here was done in my first-semester, junior-level quantum mechanics course.
Feel free to reach out if you have questions (gpassante at fullerton dot edu).
 Entanglement
Entanglement
Superposition of states in quantum mechanics is hard to think about classically - what does it mean to be both "0 and 1" (or "up" and "down", "alive" and "dead")? Many questions related to the interpretation of such a state (is this a statement about reality, or about our knowledge? Is there a difference?) were treated as philosophical for a long time. After Bell's theoretical work and then following experimental (Nobel prize-winning) efforts, we now have answers to some of these questions.
In this module, we provide an introduction to some "spooky" aspects of quantum mechanics arising from 2-particle entanglement. We work in the context set up by Einstein, Podolsky and Rosen, the so-called "EPR Paradox," and conclude with an example of a Bell inequality and an experimental test for hidden variables.
In our courses, this module takes 1 week to cover.
 Notes for Faculty
Notes for Faculty
We have written brief notes on the topics in this module that are intended for faculty adopters.
Browse below, or download all as a single pdf file (see Materials Download section below).
Topics:
The experimental context for this module is a gedanken experiment (since realized in the lab) building on ideas from Einstein, and outlined by David Mermin in a 1985 Physics Today article: "Is the Moon There when Nobody Looks?" It is described in the language of 2-particle entangled states of spin-1/2 objects. The setup is designed so two distant observers make simultaneous measurements on their particles (each part of an entangled pair), and the correlations of outcomes they measure would lead Einstein (or anyone believing that widely separated particles cannot influence each other) to argue that the measurable physical properties (outcomes) must already be there BEFORE a measurement is made. Einstein, Podolsky, and Rosen called these "elements of reality." We now know that experiments disagree with this - and indeed, the non-classical outcomes seem to imply "spooky action at a distance."
Prerequisites for students:
The required formalism is largely described in the sections below, but to follow the notation, students need to have some prerequisite skills, including familiarity with Dirac notation for single-particle states, e.g. $\ket{+}$ for a spin-up state (in the Z-basis). (Through this section, we may casually switch notations e.g. to $\ket{\uparrow}$ or $\ket{0}$, if this makes a particular question easier to state or discuss.)
In the downloadable lecture notes, we make use of a setup where measurement of a spin-1/2 object can be made along an arbitrary axis pointing in the $\hat{n}$ direction. We work with measurements constrained to the x-z plane. Students are thus expected to know the following (and if not, you will need to teach this before this unit):
- A spin-1/2 object polarized in the $+\hat{n}$ direction (in the x-z plane, tilted by angle $\theta$ from the $z$-axis) can be written:
$\ket{+}_n = \cos\frac{\theta}{2} \ket{+} + \sin\frac{\theta}{2} \ket{-}$ - The probability that a single spin-1/2 particle in any quantum state $\ket{\psi}$ will be measured as "up" in the $\hat{n}$ direction is thus given by $| {}_n\bra{+} \ket{\psi}|^2$.
Physicists continue to debate "interpretation" of quantum states, but this broader module is designed to introduce students to Bell tests and to present the experimental evidence that shows entangled states do not imply "local hidden variables" (or what Einstein called "elements of reality" in his EPR paper).
We thus begin our unit on entanglement (leading to an understanding of the EPR paradox) with a brief discussion of how one interprets a superposition state as philosophically distinct from a mixed state. We do not go into the mathematics of mixed states, as it is not needed for our discussion.
Both superposition and mixed states yield definite probabilities for measurement outcomes, but they are not the same: superposition states contain relative phase information which can lead to distinct experimental outcomes. (In a very simplistic way, one might say that a superposition is "this AND that" while a mixed state is "this OR that" with given probabilities. )
This section is also a good time to talk about the different language you are using to describe different states and what is interpretation vs experimental outcome.
To talk about entanglement, we need to introduce the notation for referring to two particles. We use the tensor product ($\otimes$) to 'join' two states (and also to join two Hilbert spaces).
The state $\ket{0} \otimes \ket{1} = \ket{01}$ (note that we often suppress the $\otimes$ symbol) is a two-particle state where the first particle is in the state $\ket{0}$ and the second particle is in the state $\ket{1}$.
Two-particle states that can be written as $\ket{\psi} \otimes \ket{\phi}$ are straightforward. For example, we can have $\frac{1}{\sqrt{2}} ( \ket{0} - \ket{1}) \otimes \frac{1}{\sqrt{3}}(\ket{0} + \sqrt{2} \ket{1})$ that can be expanded out as $\frac{1}{\sqrt{6}} ( \ket{00} + \sqrt{2}\ket{01} - \ket{10} - \sqrt{2} \ket{11})$.
Entangled states are defined as states that CANNOT be written as $\ket{\psi} \otimes \ket{\phi}$.
An example of an entangled state is $\frac{1}{\sqrt{2}} (\ket{00} + \ket{11})$. The example concept tests below help show the weirdness of entangled states.
Consider an experiment with a spin-0 source producing pairs of spin-1/2 particles in quantum state $\ket{\psi} = \frac{1}{\sqrt{2}}(\ket{+-} - \ket{-+})$. This state (or equivalently, simply the fact that we start with a spin-0 system and must conserve total angular momentum) ensures that any measurement by observer #1 of the component of spin along any given direction will be perfectly anticorrelated with a measurement by observer #2 in the same direction.
How can we explain such perfect anticorrelation for well-separated observers?
A natural idea is that the particles must carry with them information about what outcome will be measured from the state. This is referred to as "local hidden variables". Einstein argued in his 1935 EPR paper that such information (or "elements of reality") must exist in order to explain perfect anticorrelations if the observers are very far apart. Otherwise, such anticorrelation would have to be an (absurd?) "spooky action at a distance".
In a highly simplified Gedanken experiment, as shown below, the observers agree to make only one of 3 choices for axis direction, axes $\hat{a}$, $\hat{b}$, or $\hat{c}$, oriented $120^{\circ}$ apart (all in the plane of the page.) The decision of what direction to measure along is made randomly, at the same time, immediately before the measurement. This is done to ensure that observers #1 and #2 cannot communicate to each other what their measurement direction choices are.

(Note: directions ($\hat{a}$, $\hat{b}$, $\hat{c}$) all lie in the plane of the page, it is easy to incorrectly misinterpret the diagram above as showing 3-D right handed coordinates)
The existence of local hidden variables would mean that each particle carries an instruction set of what would be measured along any of these three direction choices. In this experiment, with $\ket{\psi} = \frac{1}{\sqrt{2}}(\ket{+-} - \ket{-+})$, the two particles must have "opposite" instruction sets to ensure perfect anticorrelation. These hidden variables need to exist before any measurement is made and include instructions for measurements along all measurement directions.
The experiment repeats many times, and the outcome ($+$ or $-$) for each particle is recorded (but not the axis of measurement). We compute the probability after many trials that the two observers record the SAME outcome (both $+$ or both $-$). By enumerating all possibilities, we quickly establish that Prob(same) $\le$ 4/9, no matter what the distribution of "hidden instruction sets" might be. This is a Bell inequality; it arises simply from counting. It must hold for any local hidden-variable theory.
More details are found in the next section and our downloadable notes.
References: The original EPR paper is quite readable (Phys. Rev. 47, 777 (1935)), but we find the suggested experimental context of position and momentum harder for our students than the spin version. The setup we use follows Mermin's (excellent) paper "Is the moon there when nobody looks?"
The previous section on Hidden Variables set up an example of a "Bell test." An initial 2-particle state $\ket{\psi} = \frac{1}{\sqrt{2}}(\ket{+-} - \ket{-+})$ is created, and well-separated observers Alice and Bob each measure one of the two particles. They choose to measure spin along one of three randomly-selected axes, $120^{\circ}$ apart. Each individual measurement is recorded only as $+$ or $-$ (they do not record the axis measured). After many measurements, they compare outcomes and determine Probability(same outcome).
A quantum calculation begins by computing Prob($++$) in the special case that observer #1 measures in the z-direction, and observer #2 is in any of the (three) $\hat{n}$ directions:
$Prob(\#1 \ is\ +\hat{z}, \#2\ is +\hat{n}) = |\ \bra{+}\ \bra{+_n}\ \ket{\psi}\ |^2$
Using $\ket{+_n} = \cos{\theta/2}\ket{+} + \sin{\theta/2} \ket{-}$, we find this probability is $\frac{1}{2}\sin^2{\theta/2}$ (see lecture notes for more details) Adding Prob(--) = Prob(++), we get (for this situation):
Prob(same) = $\sin^2{\theta/2}$ .
Averaging over the other possibilities for measurement combinations (e.g. that $\hat{n}$ can be oriented at $\theta = 0, \pm 120^{\circ}$ with equal likelihood), we conclude Prob(same) = 0.5
This outcome has been well-tested experimentally. This violation of the "Bell inequality" (Prob(Same) $\le$ 4/9) experimentally validates quantum theory and is explicitly inconsistent with local hidden variable theories.
Reference:
"Significant-Loophole-Free Test of Bell’s Theorem with Entangled Photons ", Marissa Giustina et al., Phys. Rev. Lett. 115, 250401 (2015)
 Material Downloads
Material Downloads
We have a set of clicker questions and homework questions that can be adapted for use in your classroom, including transitioning some questions to group whiteboard activities or group exams.
Our classroom implementations of these materials, with our lecture notes and the set of clicker questions, homework, and exam questions we used are found in the "DOWNLOAD ALL" zip file below.
- Concept tests for Entanglement and EPR


- Homework for Entanglement and EPR


- Exam questions for Entanglement and EPR


- Tutorial on Entanglement and EPR


- Notes for Faculty


- DOWNLOAD ALL (including our implementations)


(For more information on how we implement these types of materials in our upper-division classes, see our related AJP paper.)
Quick link to relevant AcePhysics.net Tutorial (which can be done in class, alone or in groups, or at home alone)
- EPR and entangled states - This tutorial has questions about spin-0 Bell states and entanglement. It is framed in a context of cryptography, but does not assume any class instruction or background on quantum cryptography.
If you are assigning this activity in your course, email us at hello[at]acephysics[dot]net to get a course page where you can access student completion information.
 Online Resources
Online Resources
Quick link to relevant AcePhysics.net Tutorial (which can be done in class, alone or in groups, or at home alone)
- EPR and entangled states - This tutorial has questions about spin-0 Bell states and entanglement. It is framed in a context of cryptography, but does not assume any class instruction or background on quantum cryptography.
If you are assigning this activity in your course, email us at hello[at]acephysics[dot]net to get a course page where you can access student completion information.
For more information on how we implement these types of materials in our upper-division classes, see our related AJP paper.
Explore other topics within QIS for QM
Introductory Quantum Information Science Materials
Who is this for? These materials are for anyone teaching an introductory (first course in) quantum computing or quantum information science.
Why did we create these materials?
- To assist faculty aiming to incorporate more active learning elements into their classes.
- To support students from multiple different academic backgrounds (physics, computer science, engineering, math, chemistry,...)
Why these topics? We focus on just a few fundamental ideas in QIS. Some students may find these materials too easy, while others may find them too hard. That is exactly the reason for their existence! The goal is to provide students with deep conceptual practice, allowing all students to reach a level of understanding that will set the foundation for future QIS learning.
What will you find here?
- Links to free online tutorials (interactive worksheets that provide students with real-time feedback as they practice, suitable for homework or in-class group work.
- Paper versions of those same tutorials, for use in class
- Clicker questions (conceptual questions that can be used to make your lectures more interactive)
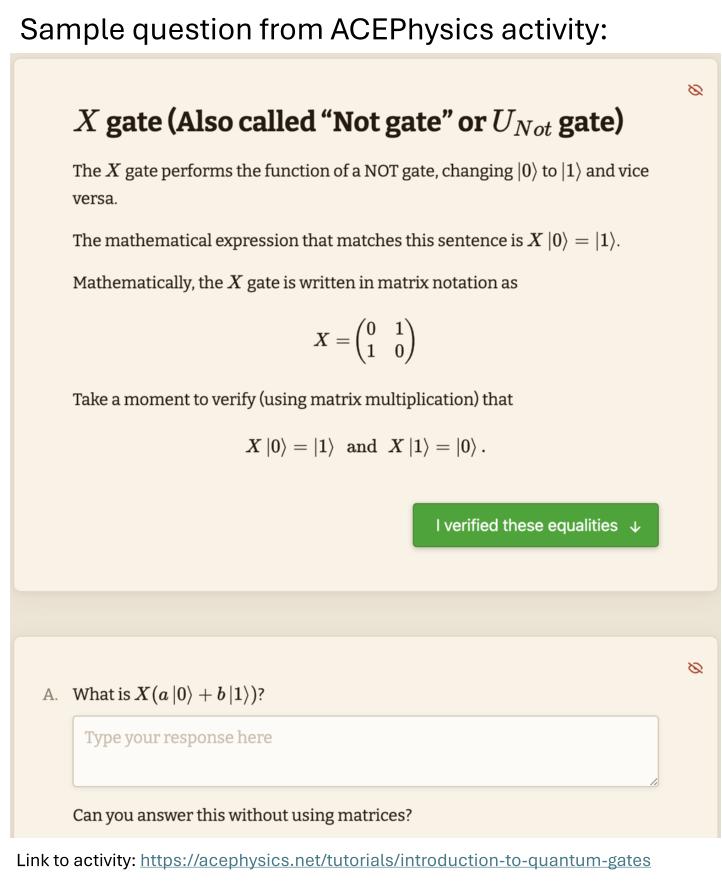
 Introduction to Quantum Gates
Introduction to Quantum Gates
Content: Superposition states, single-qubit gates (X, Z, H, and I), Dirac and matrix notation, measurement probabilities, and single-qubit X, Z, H, and I gates.
Prerequisite knowledge: None. However, previous classroom introduction to basic ideas and notation of quantum states (including Dirac and matrix notation) is useful.
Faculty notes: See the first 4 entries in "Notes for Faculty" in our QIS for QM tab labeled "Qubits, Quantum Gates, ...": (1. Motivation, 2. Qubits, 3. Parts of a Computer, and 4. Quantum Gates)
Materials available:
- AcePhysics online interactive tutorial - use this link:
acephysics.net/tutorials/introduction-to-quantum-gates - Paper worksheet: Paper version of the online tutorial for use in class with instructor supervision. (Download below, along with instructor notes for facilitation)
- "Clicker questions:" Editable in-class conceptual questions with instructor notes in PowerPoint format. (Download below)
- 1. Screenshot of online activity

- 2a. Introduction to Quantum Gates (paper version)


- 2b. Instructor's Guide to paper version


- 3. Clicker questions - Intro to Quantum Gates


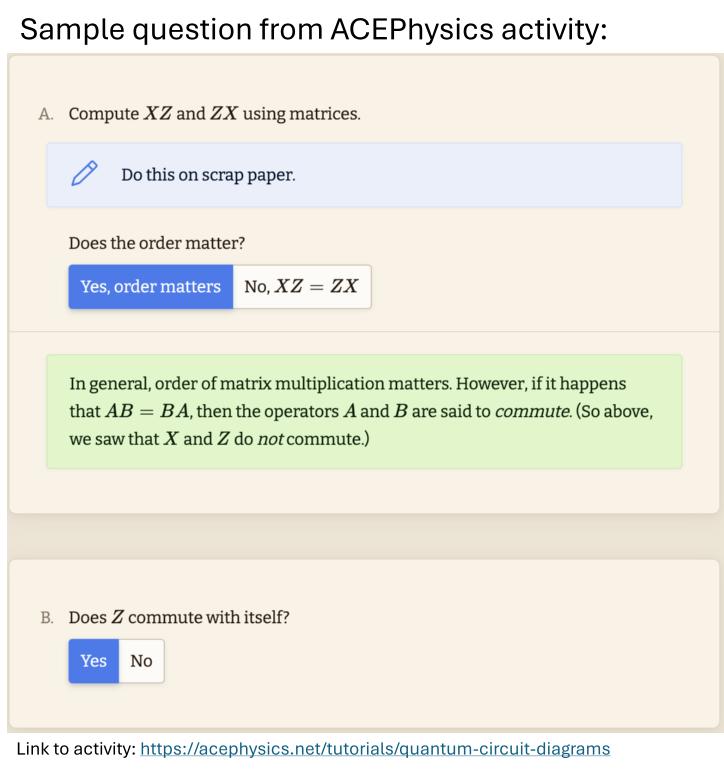
 Quantum Circuit Diagrams
Quantum Circuit Diagrams
Content: Practice with single-qubit gates represented as circuit diagrams
Prerequisite knowledge: Basic gates and quantum states. (This can all be found in the previous tutorial, "Introduction to Quantum Gates.")
Faculty notes: See the fifth entry under "Notes for Faculty" in our QIS for QM tab labeled "Qubits, Quantum Gates, ...": (5. Circuit Diagrams)
Materials available:
- AcePhysics online interactive tutorial - use this link:
acephysics.net/tutorials/quantum-circuit-diagrams - Paper worksheet: Paper version of the online tutorial for use in class with instructor supervision. (Download below, along with instructor notes for facilitation)
- "Clicker questions:" Editable in-class conceptual questions with instructor notes in PowerPoint format. (Download below.)
 Tensor Products
Tensor Products
Content: Helping students describe systems with multiple qubits
Prerequisite knowledge: Students should be familiar with quantum states, basic single-qubit gates and circuit diagram conventions. (i.e., The sections above, on this page.)
Faculty notes: See the sixth entry under "Notes for Faculty" in our QIS for QM tab labeled "Qubits, Quantum Gates, ..." : (6. Tensor products of states and operators)
Materials available:
- AcePhysics online interactive tutorial - use this link:
acephysics.net/tutorials/tensor-products - Paper worksheet: Paper version of the online tutorial for use in class with instructor supervision. (Download below, along with instructor notes for facilitation)
 CNOT and entanglement
CNOT and entanglement
Content: An introduction to the controlled-NOT (CNOT) gate and the related concept of entanglement.
Prerequisite knowledge: Students should be familiar with quantum states, basic single-qubit gates and circuit diagram conventions, and the tensor product. (i.e., The previous sections on this page.)
Faculty notes: See entries 7-8 under "Notes for Faculty" in our QIS for QM tab labeled "Qubits, Quantum Gates, ...": (7. Definition of Entanglement and 8. Entangling gates/CNOT)
Materials available:
- AcePhysics online interactive tutorial - use this link:
acephysics.net/tutorials/cnot-entanglement - Paper worksheet: Paper version of the online tutorial for use in class with instructor supervision. (Download below, along with instructor notes for facilitation)
 Quantum Cryptography (BB84)
Quantum Cryptography (BB84)
Content: This activity takes students through the BB84 quantum key distribution protocol. It uses quantum circuit notation and includes the effect of an eavesdropper.
Prerequisite knowledge: Basic single qubit gates (in particular, the Hadamard, H gate). Students should know how to predict probabilistic outcomes of measurements in the computational (Z) basis on superpositions of $|0\rangle, |1\rangle, |+\rangle, |-\rangle$ states.
Faculty notes: See the "Notes for Faculty" in our QIS for QM tab: Quantum Cryptography
Materials available:
- AcePhysics online interactive tutorial - use this link:
acephysics.net/tutorials/quantum-cryptography - Paper worksheet: Paper version of the online tutorial for use in class with instructor supervision. (Download below, along with instructor notes for facilitation)
What if I need help implementing active learning in my class?
Physport.org has some short guides to help you facilitate in-class tutorials, as well as to help you implement clicker questions in lectures effectively.)
Conceptual Assessment for Quantum Computing (QCCS)
|
We have developed a 20-question conceptual assessment that targets the introductory content in a quantum computing course (known as the QCCS). The survey is not designed to assess individual students but rather the course as a whole. The general content covered in the assessment includes mathematical foundations, superposition and measurement, quantum gates and circuit diagrams, and entanglement. The questions are conceptual in nature (not computational). Want to give the assessment to your students? |
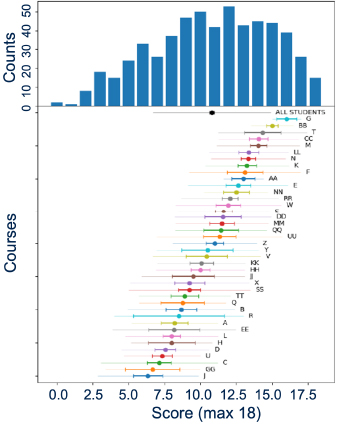 |
| Figure: Aggregated results for 568 students in a beta test of the QCCS across 40 different courses. Averages for each course is shown below the histogram, we see wide variation across classes, populations and pedagogical approaches. |
Who we are
We are PER research faculty teaching at very different institutions - different in class sizes and setups, student demographics, institutional research-focus - but all interested in helping introduce undergraduates to basic elements of Quantum Information Science. We have all taught a variety of quantum courses for many years.
The materials you will find here are not meant to be taken as givens, this is not a "fixed curriculum" that you are supposed to fully adopt (or reject). We hope that you will be inspired by some of the activities, notes, concept-tests, homeworks and more, and will borrow and adapt them for your own situation and students. We do not all use exactly the same materials ourselves.
We have borrowed where we can from PER literature on Quantum Mechanics (and tried - but apologize up front where we occasionally have failed - to appropriately credit the the hard development work of others!) We do not claim that these materials are "Research-validated" (they are still under development), but cheerfully present sometimes half-baked or partially-tested materials that we might argue are "research-based", a vaguer but perhaps more realistic description.
We welcome feedback and suggestions. If you make significant changes or additions, and particularly if you have classroom evidence that suggests it works well - let us know. We hope someday this site will be flexible enough to allow for community-sharing of new resources, and will work towards making that happen. (See contact information below.)
 |
Gina Passante is Associate Professor of Physics and Director of the Catalyst Center for the Advancement of Research in Teaching and Learning Math and Science at Cal State University Fullerton. She received her Ph.D. in quantum computing at U. Waterloo before transitioning to PER. |
 |
Steve Pollock is a Physics professor at CU Boulder who has engaged in PER for 20+ years. He has a background in theoretical nuclear physics. He is an APS Fellow, and was named US Professor of the Year in 2013. His PER work is focused on assessment and curricular materials development in upper-division physics courses. Please email if you are interested in teaching materials for other courses (including middle division Classical Mechanics, E&M, and others). He can be reached at Steven.Pollock (at) Colorado.edu |
 |
Bethany Wilcox is a Physics professor at CU Boulder. Her PER work focuses on the development of research-based and validated assessments of student learning that can be used to measure the impact of curricular changes or compare student learning across courses and institutions. In particular, she is utilizing advanced testing theories to explore viable options for creating modular assessments that can address variations in content coverage in across courses. |
We acknowledge the hard work of our students, including Josephine Meyer (University of Colorado Boulder), Jonan-Rohi Plueger (University of Colorado Boulder), and Bianca Cervantes (former California State University, Fullerton).
We are funded in part by NSF DUE- 2012147 and 2011958: Collaborative Research: Connecting Spins-First Quantum Mechanics Instruction to Quantum Information Science
PLEASE USE AND ADAPT whatever is helpful to you, however it will most benefit your students. Please credit our work if you share your materials beyond your own classes. Please make an effort to keep assessment materials off the open web - alter questions for your students.

 Qubits, Quantum Gates, and Teleportation
Qubits, Quantum Gates, and Teleportation Quantum Cryptography
Quantum Cryptography